7.10 Wave Pack­ets

This sec­tion gives a full de­scrip­tion of the mo­tion of a par­ti­cle ac­cord­ing to quan­tum me­chan­ics. It will be as­sumed that the par­ti­cle is in free space, so that the po­ten­tial en­ergy is zero. In ad­di­tion, to keep the analy­sis con­cise and the re­sults easy to graph, it will be as­sumed that the mo­tion is only in the $x$-​di­rec­tion. The re­sults may eas­ily be ex­tended to three di­men­sions by us­ing sep­a­ra­tion of vari­ables.

One thing that the analy­sis will show is how lim­it­ing the un­cer­tainty in both mo­men­tum and po­si­tion pro­duces the var­i­ous fea­tures of clas­si­cal New­ton­ian mo­tion. It may be re­called that in New­ton­ian mo­tion through free space, the lin­ear mo­men­tum $p$ is con­stant. In ad­di­tion, since $p$$\raisebox{.5pt}{$/$}$​$m$ is the ve­loc­ity $v$, the clas­si­cal par­ti­cle will move at con­stant speed. So clas­si­cal New­ton­ian mo­tion would say:

$$v = \frac{p}{m} = \mbox{constant} \qquad x = v t + x_0\qquad \mbox{for Newtonian motion in free space}$$

(Note that $p$ is used to in­di­cate $p_x$ in this and the fol­low­ing sec­tions.)

7.10.1 So­lu­tion of the Schrö­din­ger equa­tion.

As dis­cussed in sec­tion 7.1, the un­steady evo­lu­tion of a quan­tum sys­tem may be de­ter­mined by find­ing the eigen­func­tions of the Hamil­ton­ian and giv­ing them co­ef­fi­cients that are pro­por­tional to $e^{-{{\rm i}}Et/\hbar}$. This will be worked out in this sub­sec­tion.

For a free par­ti­cle, there is only ki­netic en­ergy, so in one di­men­sion the Hamil­ton­ian eigen­value prob­lem is:

$$-\frac{\hbar^2}{2m} \frac{\partial^2 \psi}{\partial x^2} = E \psi$$ (7.56)

So­lu­tions to this equa­tion take the form of ex­po­nen­tials

$$\psi_E = A e^{\pm{\rm i}\sqrt{2mE} x/\hbar}$$

where $A$ is a con­stant.

Note that $E$ must be pos­i­tive: if the square root would be imag­i­nary, the so­lu­tion would blow up ex­po­nen­tially at large pos­i­tive or neg­a­tive $x$. Since the square mag­ni­tude of $\psi$ at a point gives the prob­a­bil­ity of find­ing the par­ti­cle near that po­si­tion, blow up at in­fin­ity would im­ply that the par­ti­cle must be at in­fin­ity with cer­tainty.

The en­ergy eigen­func­tion above is re­ally the same as the eigen­func­tion of the $x$-​mo­men­tum op­er­a­tor ${\widehat p}_x$ de­rived in the pre­vi­ous sec­tion:

$$\psi_E = \frac{1}{\sqrt{2\pi\hbar}} e^{{\rm i}p x/\hbar} \quad \mbox { with } p=\pm \sqrt{2mE}$$ (7.57)

The rea­son that the mo­men­tum eigen­func­tions are also en­ergy eigen­func­tions is that the en­ergy is all ki­netic en­ergy, and the ki­netic op­er­a­tor equals ${\widehat T}$ $\vphantom0\raisebox{1.5pt}{$=$}$ ${\widehat p}^2$$\raisebox{.5pt}{$/$}$​$2m$. So eigen­func­tions with pre­cise mo­men­tum $p$ have pre­cise en­ergy $p^2$$\raisebox{.5pt}{$/$}$​$2m$.

As shown by (7.55) in the pre­vi­ous sec­tion, com­bi­na­tions of mo­men­tum eigen­func­tions take the form of an in­te­gral rather than a sum. In the one-di­men­sion­al case that in­te­gral is:

$$\Psi(x,t)= \frac{1}{\sqrt{2\pi\hbar}} \int_{-\infty}^\infty \Phi(p,t) e^{{\rm i}p x/\hbar} {\,\rm d}p$$

where $\Phi(p,t)$ is called the mo­men­tum space wave func­tion.

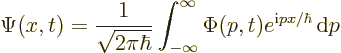
Whether a sum or an in­te­gral, the Schrö­din­ger equa­tion still re­quires that the co­ef­fi­cient of each en­ergy eigen­func­tion varies in time pro­por­tional to $e^{-{{\rm i}}Et/\hbar}$. The co­ef­fi­cient here is the mo­men­tum space wave func­tion $\Phi$, and the en­ergy is $E$ $\vphantom0\raisebox{1.5pt}{$=$}$ $p^2$$\raisebox{.5pt}{$/$}$​$2m$, so the so­lu­tion of the Schrö­din­ger equa­tion must be:

$$\fbox{$\displaystyle \Psi(x,t)= \frac{1}{\sqrt{2\pi\hbar}}... ...\frac{p}{{\scriptscriptstyle 2}m}} t)/\hbar} {\,\rm d}p $} %$$ (7.58)

Here $\Phi_0(p)\equiv\Phi(p,0)$ is de­ter­mined by what­ever ini­tial con­di­tions are rel­e­vant to the sit­u­a­tion that is to be de­scribed. The above in­te­gral is the fi­nal so­lu­tion for a par­ti­cle in free space.

Key Points

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

In free space, mo­men­tum eigen­func­tions are also en­ergy eigen­func­tions.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The one-di­men­sion­al wave func­tion for a par­ti­cle in free space is given by (7.58).

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The func­tion $\Phi_0$ is still to be cho­sen to pro­duce what­ever phys­i­cal sit­u­a­tion is to be de­scribed.

7.10.2 Com­po­nent wave so­lu­tions

Be­fore try­ing to in­ter­pret the com­plete ob­tained so­lu­tion (7.58) for the wave func­tion of a par­ti­cle in free space, it is in­struc­tive first to have a look at the com­po­nent so­lu­tions, de­fined by

$$\psi_{\rm {w}} \equiv e^{{\rm i}p (x - {\textstyle\frac{p}{{\scriptscriptstyle 2}m}} t)/\hbar}$$ (7.59)

These so­lu­tions will be called com­po­nent waves; both their real and imag­i­nary parts are si­nu­soidal, as can be seen from the Euler for­mula (2.5).

$$\psi_{\rm {w}} = \cos\left(p \Big(x - \frac{p}{2m} t\Big)/... ... {\rm i}\sin\left(p \Big(x - \frac{p}{2m} t\Big)/\hbar\right)$$

In fig­ure 7.11, the real part of the wave (in other words, the co­sine), is sketched as the red curve; also the mag­ni­tude of the wave (which is unity) is shown as the top black line, and mi­nus the mag­ni­tude is drawn as the bot­tom black line.

Fig­ure 7.11: The real part (red) and en­ve­lope (black) of an ex­am­ple wave.

The black lines en­close the real part of the wave, and will be called the en­ve­lope. Since their ver­ti­cal sep­a­ra­tion is twice the mag­ni­tude of the wave func­tion, the ver­ti­cal sep­a­ra­tion be­tween the black lines at a point is a mea­sure for the prob­a­bil­ity of find­ing the par­ti­cle near that point.

The con­stant sep­a­ra­tion be­tween the black lines shows that there is ab­solutely no lo­cal­iza­tion of the par­ti­cle to any par­tic­u­lar re­gion. The par­ti­cle is equally likely to be found at every point in the in­fi­nite range. This also graph­i­cally demon­strates the nor­mal­iza­tion prob­lem of the mo­men­tum eigen­func­tions dis­cussed in the pre­vi­ous sec­tion: the to­tal prob­a­bil­ity of find­ing the par­ti­cle just keeps get­ting big­ger and big­ger, the larger the range you look in. So there is no way that the to­tal prob­a­bil­ity of find­ing the par­ti­cle can be lim­ited to one as it should be.

The rea­son for the com­plete lack of lo­cal­iza­tion is the fact that the com­po­nent wave so­lu­tions have an ex­act mo­men­tum $p$. With zero un­cer­tainty in mo­men­tum, Heisen­berg's un­cer­tainty re­la­tion­ship says that there must be in­fi­nite un­cer­tainty in po­si­tion. There is.

There is an­other funny thing about the com­po­nent waves: when plot­ted for dif­fer­ent times, it is seen that the real part of the wave moves to­wards the right with a speed $p$$\raisebox{.5pt}{$/$}$​$2m$ $\vphantom0\raisebox{1.5pt}{$=$}$ $\frac12v$, as il­lus­trated in fig­ure 7.12.

Fig­ure 7.12: The wave moves with the phase speed.

 

Move your mouse over the fig­ure to see the an­i­ma­tion. Javascript must be en­abled on your browser. Give it a few sec­onds for the an­i­ma­tion to load, es­pe­cially on a phone line.

This is un­ex­pected, be­cause clas­si­cally the par­ti­cle moves with speed $v$, not $\frac12v$. The prob­lem is that the speed with which the wave moves, called the “phase speed,” is not mean­ing­ful phys­i­cally. In fact, with­out any­thing like a lo­ca­tion for the par­ti­cle, there is no way to de­fine a phys­i­cal ve­loc­ity for a com­po­nent wave.

Key Points

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

Com­po­nent waves pro­vide no lo­cal­iza­tion of the par­ti­cle at all.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

Their real part is a mov­ing co­sine. Sim­i­larly their imag­i­nary part is a mov­ing sine.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The speed of mo­tion of the co­sine or sine is half the speed of a clas­si­cal par­ti­cle with that mo­men­tum.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

This speed is called the phase speed and is not rel­e­vant phys­i­cally.

7.10.3 Wave pack­ets

As Heisen­berg’s prin­ci­ple in­di­cates, in or­der to get some lo­cal­iza­tion of the po­si­tion of a par­ti­cle, some un­cer­tainty must be al­lowed in mo­men­tum. That means that you must take the ini­tial mo­men­tum space wave func­tion $\Phi_0$ in (7.58) to be nonzero over at least some small in­ter­val of dif­fer­ent mo­men­tum val­ues $p$. Such a com­bi­na­tion of com­po­nent waves is called a wave packet.

The wave func­tion for a typ­i­cal wave packet is sketched in fig­ure 7.13. The red line is again the real part of the wave func­tion, and the black lines are the en­ve­lope en­clos­ing the wave; they equal plus and mi­nus the mag­ni­tude of the wave func­tion.

Fig­ure 7.13: The real part (red) and mag­ni­tude or en­ve­lope (black) of a wave packet. (Schematic).

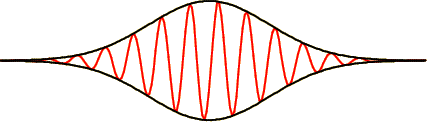The ver­ti­cal sep­a­ra­tion be­tween the black lines is again a mea­sure of the prob­a­bil­ity of find­ing the par­ti­cle near that lo­ca­tion. It is seen that the pos­si­ble lo­ca­tions of the par­ti­cle are now re­stricted to a fi­nite re­gion, the re­gion in which the ver­ti­cal dis­tance be­tween the black lines is nonzero.

If the en­ve­lope changes lo­ca­tion with time, and it does, then so does the re­gion where the par­ti­cle can be found. This then fi­nally is the cor­rect pic­ture of mo­tion in quan­tum me­chan­ics: the re­gion in which the par­ti­cle can be found prop­a­gates through space.

The lim­it­ing case of the mo­tion of a macro­scopic New­ton­ian point mass can now be bet­ter un­der­stood. As noted in sec­tion 7.2.1, for such a par­ti­cle the un­cer­tainty in po­si­tion is neg­li­gi­ble. The wave packet in which the par­ti­cle can be found, as sketched in fig­ure 7.13, is so small that it can be con­sid­ered to be a point. To that ap­prox­i­ma­tion the par­ti­cle then has a point po­si­tion, which is the nor­mal clas­si­cal de­scrip­tion.

The clas­si­cal de­scrip­tion also re­quires that the par­ti­cle moves with ve­loc­ity $u$ $\vphantom0\raisebox{1.5pt}{$=$}$ $p$$\raisebox{.5pt}{$/$}$​$m$, which is twice the speed $p$$\raisebox{.5pt}{$/$}$​$2m$ of the wave. So the en­ve­lope should move twice as fast as the wave. This is in­di­cated in fig­ure 7.14 by the length of the bars, which show the mo­tion of a point on the en­ve­lope and of a point on the wave dur­ing a small time in­ter­val.

Fig­ure 7.14: The ve­loc­i­ties of wave and en­ve­lope are not equal.

 

Move your mouse over the fig­ure to see the an­i­ma­tion. Javascript must be en­abled on your browser. Give it a few sec­onds for the an­i­ma­tion to load, es­pe­cially on a phone line.

That the en­ve­lope does in­deed move at speed $p$$\raisebox{.5pt}{$/$}$​$m$ can be seen if you de­fine the rep­re­sen­ta­tive po­si­tion of the en­ve­lope to be the ex­pec­ta­tion value of po­si­tion. That po­si­tion must be some­where in the mid­dle of the wave packet. The ex­pec­ta­tion value of po­si­tion moves ac­cord­ing to Ehren­fest's the­o­rem of sec­tion 7.2.1 with a speed $\left\langle{p}\right\rangle$$\raisebox{.5pt}{$/$}$​$m$, where $\left\langle{p}\right\rangle$ is the ex­pec­ta­tion value of mo­men­tum, which must be con­stant since there is no force. Since the un­cer­tainty in mo­men­tum is small for a macro­scopic par­ti­cle, the ex­pec­ta­tion value of mo­men­tum $\left\langle{p}\right\rangle$ can be taken to be the mo­men­tum $p$.

Key Points

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

A wave packet is a com­bi­na­tion of waves with about the same mo­men­tum.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

Com­bin­ing waves into wave pack­ets can pro­vide lo­cal­iza­tion of par­ti­cles.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The en­ve­lope of the wave packet shows the re­gion where the par­ti­cle is likely to be found.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

This re­gion prop­a­gates with the clas­si­cal par­ti­cle ve­loc­ity.

7.10.4 Group ve­loc­ity

As the pre­vi­ous sub­sec­tion ex­plained, par­ti­cle mo­tion in clas­si­cal me­chan­ics is equiv­a­lent to the mo­tion of wave pack­ets in quan­tum me­chan­ics. Mo­tion of a wave packet im­plies that the re­gion in which the par­ti­cle can be found changes po­si­tion.

Mo­tion of wave pack­ets is not just im­por­tant for un­der­stand­ing where par­ti­cles in free space end up. It is also crit­i­cal for the quan­tum me­chan­ics of for ex­am­ple solids, in which elec­trons, pho­tons, and phonons (quanta of crys­tal vi­bra­tions) move around in an en­vi­ron­ment that is clut­tered with other par­ti­cles. And it is also of great im­por­tance in clas­si­cal ap­pli­ca­tions, such as acoustics in solids and flu­ids, wa­ter waves, sta­bil­ity the­ory of flows, elec­tro­mag­ne­to­dy­nam­ics, etcetera. This sec­tion ex­plains how wave pack­ets move in such more gen­eral sys­tems. Only the one-di­men­sion­al case will be con­sid­ered, but the gen­er­al­iza­tion to three di­men­sions is straight­for­ward.

The sys­tems of in­ter­est have com­po­nent wave so­lu­tions of the gen­eral form:

$$\fbox{$\displaystyle \mbox{component wave:}\quad \psi_{\rm{w}} = e^{{\rm i}(kx - \omega t)} $} %$$ (7.60)

The con­stant $k$ is called the “wave num­ber,” and $\omega$ the “an­gu­lar fre­quency.” The wave num­ber and fre­quency must be real for the analy­sis in this sec­tion to ap­ply. That means that the mag­ni­tude of the com­po­nent waves must not change with space nor time. Such sys­tems are called nondis­si­pa­tive: al­though a com­bi­na­tion of waves may get dis­persed over space, its square mag­ni­tude in­te­gral will be con­served. (This is true on ac­count of Par­se­val’s re­la­tion, {A.26}.)

For a par­ti­cle in free space ac­cord­ing to the pre­vi­ous sub­sec­tion:

$$k=\frac{p}{\hbar} \qquad \omega = \frac{p^2}{2m\hbar}$$

There­fore, for a par­ti­cle in free space the wave num­ber $k$ is just a rescaled lin­ear mo­men­tum, and the fre­quency $\omega$ is just a rescaled ki­netic en­ergy. This will be dif­fer­ent for a par­ti­cle in a non­triv­ial sur­round­ings.

Re­gard­less of what kind of sys­tem it is, the re­la­tion­ship be­tween the fre­quency and the wave num­ber is called the

$$\fbox{$\displaystyle \mbox{dispersion relation:}\quad \omega = \omega(k) $} %$$ (7.61)

It re­ally de­fines the physics of the wave prop­a­ga­tion.

Since the waves are of the form $e^{{{\rm i}}k(x-\frac{\omega}{k}t)}$, the wave is con­stant if $x$ $\vphantom0\raisebox{1.5pt}{$=$}$ $(\omega/k)t$ plus any con­stant. Such points move with the

$$\fbox{$\displaystyle \mbox{phase velocity:}\quad v_{\rm{p}} \equiv \frac{\omega}{k} $} %$$ (7.62)

In free space, the phase ve­loc­ity is half the clas­si­cal ve­loc­ity.

How­ever, as noted in the pre­vi­ous sub­sec­tion, wave pack­ets do not nor­mally move with the phase ve­loc­ity. The ve­loc­ity that they do move with is called the group ve­loc­ity. For a par­ti­cle in free space, you can in­fer that the group ve­loc­ity is the same as the clas­si­cal ve­loc­ity from Ehren­fest's the­o­rem, but that does not work for more gen­eral sys­tems. The ap­proach will there­fore be to sim­ply de­fine the group ve­loc­ity as

$$\fbox{$\displaystyle \mbox{group velocity:}\quad v_{\rm{g}} \equiv \frac{{\rm d}\omega}{{\rm d}k} $} %$$ (7.63)

and then to ex­plore how the so-de­fined group ve­loc­ity re­lates to the mo­tion of wave pack­ets.

Wave pack­ets are com­bi­na­tions of com­po­nent waves, and the most gen­eral com­bi­na­tion of waves takes the form

$$\fbox{$\displaystyle \Psi(x,t) = \frac{1}{\sqrt{2\pi}} \i... ... \overline{\Phi}_0(k) e^{{\rm i}(kx-\omega t)}{\,\rm d}k $} %$$ (7.64)

Here $\overline{\Phi}_0$ is the com­plex am­pli­tude of the waves. The com­bi­na­tion $\overline{\Phi}_0e^{-{\rm i}{\omega}t}$ is called the “Fourier trans­form” of $\Psi$. The fac­tor $\sqrt{2\pi}$ is just a nor­mal­iza­tion fac­tor that might be cho­sen dif­fer­ently in an­other book. Wave pack­ets cor­re­spond to com­bi­na­tions in which the com­plex am­pli­tude $\overline{\Phi}_0(k)$ is only nonzero in a small range of wave num­bers $k$. More gen­eral com­bi­na­tions of waves may of course al­ways be split up into such wave pack­ets.

To de­scribe the mo­tion of wave pack­ets is not quite as straight­for­ward as it may seem: the en­ve­lope of a wave packet ex­tends over a fi­nite re­gion, and dif­fer­ent points on it ac­tu­ally move at some­what dif­fer­ent speeds. So what do you take as the point that de­fines the mo­tion if you want to be pre­cise? There is a trick here: con­sider very long times. For large times, the prop­a­ga­tion dis­tance is so large that it dwarfs the am­bi­gu­ity about what point to take as the po­si­tion of the en­ve­lope.

Find­ing the wave func­tion $\Psi$ for large time is a messy ex­er­cise banned to de­riva­tion {D.44}. But the con­clu­sions are fairly straight­for­ward. As­sume that the range of waves in the packet is re­stricted to some small in­ter­val $k_1$ $\raisebox{.3pt}{$<$}$ $k$ $\raisebox{.3pt}{$<$}$ $k_2$. In par­tic­u­lar, as­sume that the vari­a­tion in group ve­loc­ity is rel­a­tively small and mo­not­o­nous. In that case, for large times the wave func­tion will be neg­li­gi­bly small ex­cept in the re­gion

$$v_{{\rm {g}}1} t < x < v_{{\rm {g}}2} t$$

(In case $v_{{\rm {g}}1}$ $\raisebox{.3pt}{$>$}$ $v_{{\rm {g}}2}$, in­vert these in­equal­i­ties.) Since the vari­a­tion in group ve­loc­ity is small for the packet, it there­fore def­i­nitely does move with the group ve­loc­ity.

It is not just pos­si­ble to say where the wave func­tion is nonzero at large times. It is also pos­si­ble to write a com­plete ap­prox­i­mate wave func­tion for large times:

$$\Psi(x,t)\sim \frac{e^{\mp{\rm i}\pi/4}}{\sqrt{\vert v_{{\r... ...^{{\rm i}(k_0x-\omega_0t)} \qquad v_{{\rm {g}}0} = \frac{x}{t}$$

Here $k_0$ is the wave num­ber at which the group speed is ex­actly equal to $x$$\raisebox{.5pt}{$/$}$​$t$, $\omega_0$ is the cor­re­spond­ing fre­quency, $v_{{\rm {g}}0}'$ is the de­riv­a­tive of the group speed at that point, and $\mp$ stands for the sign of $-v_{{\rm {g}}0}'$.

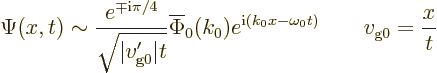
While this pre­cise ex­pres­sion may not be that im­por­tant, it is in­ter­est­ing to note that $\Psi$ de­creases in mag­ni­tude pro­por­tional to 1/$\sqrt{t}$. That can be un­der­stood from con­ser­va­tion of the prob­a­bil­ity to find the par­ti­cle. The wave packet spreads out pro­por­tional to time be­cause of the small but nonzero vari­a­tion in group ve­loc­ity. There­fore $\Psi$ must be pro­por­tional to 1$\raisebox{.5pt}{$/$}$​$\sqrt{t}$ if its square in­te­gral is to re­main un­changed.

One other in­ter­est­ing fea­ture may be de­duced from the above ex­pres­sion for $\Psi$. If you ex­am­ine the wave func­tion on the scale of a few os­cil­la­tions, it looks as if it was a sin­gle com­po­nent wave of wave num­ber $k_0$ and fre­quency $\omega_0$. Only if you look on a big­ger scale do you see that it re­ally is a wave packet. To un­der­stand why, just look at the dif­fer­en­tial

$${\rm d}(k_0x-\omega_0t)= k_0 {\rm d}x - \omega_0{\rm d}t + x{\rm d}k_0 - t{\rm d}\omega_0$$

and ob­serve that the fi­nal two terms can­cel be­cause ${\rm d}\omega_0$$\raisebox{.5pt}{$/$}$​${\rm d}{k}_0$ is the group ve­loc­ity, which equals $x$$\raisebox{.5pt}{$/$}$​$t$. There­fore changes in $k_0$ and $\omega_0$ do not show up on a small scale.

For the par­ti­cle in free space, the re­sult for the large time wave func­tion can be writ­ten out fur­ther to give

$$\Psi(x,t)\sim e^{-{\rm i}\pi/4} \sqrt{\frac{m}{t}} \Phi_0\left(\frac{mx}{t}\right) e^{{\rm i}mx^2/2\hbar t}$$

Since the group speed $p$$\raisebox{.5pt}{$/$}$​$m$ in this case is mo­not­o­nously in­creas­ing, the wave pack­ets have neg­li­gi­ble over­lap, and this is in fact the large time so­lu­tion for any com­bi­na­tion of waves, not just nar­row wave pack­ets.

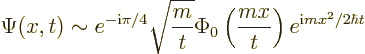
In a typ­i­cal true quan­tum me­chan­ics case, $\Phi_0$ will ex­tend over a range of wave num­bers that is not small, and may in­clude both pos­i­tive and neg­a­tive val­ues of the mo­men­tum $p$. So, there is no longer a mean­ing­ful ve­loc­ity for the wave func­tion: the wave func­tion spreads out in all di­rec­tions at ve­loc­i­ties rang­ing from neg­a­tive to pos­i­tive. For ex­am­ple, if the mo­men­tum space wave func­tion $\Phi_0$ con­sists of two nar­row nonzero re­gions, one at a pos­i­tive value of $p$ and one at a neg­a­tive value, then the wave func­tion in nor­mal space splits into two sep­a­rate wave pack­ets. One packet moves with con­stant speed to­wards the left, the other with con­stant speed to­wards the right. The same par­ti­cle is now go­ing in two com­pletely dif­fer­ent di­rec­tions at the same time. That would be un­heard of in clas­si­cal New­ton­ian me­chan­ics.

Key Points

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

Com­po­nent waves have the generic form $e^{{\rm i}(kx-{\omega}t)}$.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The con­stant $k$ is the wave num­ber.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The con­stant $\omega$ is the an­gu­lar fre­quency.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The re­la­tion be­tween $\omega$ and $k$ is called the dis­per­sion re­la­tion.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The phase ve­loc­ity is $\omega$$\raisebox{.5pt}{$/$}$​$k$. It de­scribes how fast the wave moves.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The group ve­loc­ity is ${\rm d}\omega$$\raisebox{.5pt}{$/$}$​${\rm d}{k}$. It de­scribes how fast wave pack­ets move.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

Rel­a­tively sim­ple ex­pres­sions ex­ist for the wave func­tion of wave pack­ets at large times.

7.10.5 Elec­tron mo­tion through crys­tals

One im­por­tant ap­pli­ca­tion of group ve­loc­ity is the mo­tion of con­duc­tion elec­trons through crys­talline solids. This sub­sec­tion dis­cusses it.

Con­duc­tion elec­trons in solids must move around the atoms that make up the solid. You can­not just for­get about these atoms in dis­cussing the mo­tion of the con­duc­tion elec­trons. Even semi-clas­si­cally speak­ing, the elec­trons in a solid move in a roller-coaster ride around the atoms. Any ex­ter­nal force on the elec­trons is on top of the large forces that the crys­tal al­ready ex­erts. So it is sim­ply wrong to say that the ex­ter­nal force gives mass times ac­cel­er­a­tion of the elec­trons. Only the to­tal force would do that.

Typ­i­cally, on a mi­cro­scopic scale the solid is crys­talline; in other words, the atoms are arranged in a pe­ri­odic pat­tern. That means that the forces on the elec­trons have a pe­ri­odic na­ture. As usual, any di­rect in­ter­ac­tions be­tween par­ti­cles will be ig­nored as too com­plex to an­a­lyze. There­fore, it will be as­sumed that the po­ten­tial en­ergy seen by an elec­tron is a given pe­ri­odic func­tion of po­si­tion.

It will also again be as­sumed that the mo­tion is one-di­men­sion­al. In that case the en­ergy eigen­func­tions are de­ter­mined from a one-di­men­sion­al Hamil­ton­ian eigen­value prob­lem of the form

$$-\frac{\hbar^2}{2 m_{\rm e}} \frac{\partial^2\psi}{\partial x^2} + V(x) \psi = E \psi %$$ (7.65)

Here $V(x)$ is a pe­ri­odic po­ten­tial en­ergy, with some given atomic-scale pe­riod $d$.

Three-di­men­sion­al en­ergy eigen­func­tions may be found as prod­ucts of one-di­men­sion­al ones; com­pare chap­ter 3.5.8. Un­for­tu­nately how­ever, that only works here if the three-di­men­sion­al po­ten­tial is some sum of one-di­men­sion­al ones, as in

$$V(x,y,z) = V_x(x) + V_y(y) + V_z(z)$$

That is re­ally quite lim­it­ing. The gen­eral con­clu­sions that will be reached in this sub­sec­tion con­tinue to ap­ply for any pe­ri­odic po­ten­tial, not just a sum of one-di­men­sion­al ones.

The en­ergy eigen­func­tion so­lu­tions to (7.65) take the form of Bloch waves:

$$\pp{k}/x/// = \pp{{\rm p},k}/x/// e^{{\rm i}k x} %$$ (7.66)

where $\pp{{\rm {p}},k}////$ is a pe­ri­odic func­tion of pe­riod $d$ like the po­ten­tial.

The rea­son that the en­ergy eigen­func­tions take the form of Bloch waves is not that dif­fi­cult to un­der­stand. It is a con­se­quence of the fact that com­mut­ing op­er­a­tors have com­mon eigen­func­tions, chap­ter 4.5.1. Con­sider the “trans­la­tion op­er­a­tor” ${\cal T}_d$ that shifts wave func­tions over one atomic pe­riod $d$. Since the po­ten­tial is ex­actly the same af­ter a wave func­tion is shifted over an atomic pe­riod, the Hamil­ton­ian com­mutes with the trans­la­tion op­er­a­tor. It makes no dif­fer­ence whether you ap­ply the Hamil­ton­ian be­fore or af­ter you shift a wave func­tion over an atomic pe­riod. There­fore, the en­ergy eigen­func­tions can be taken to be also eigen­func­tions of the trans­la­tion op­er­a­tor. The trans­la­tion eigen­value must have mag­ni­tude one, since the mag­ni­tude of a wave func­tion does not change when you merely shift it. There­fore the eigen­value can al­ways be writ­ten as $e^{{{\rm i}}kd}$ for some real value $k$. And that means that if you write the eigen­func­tion in the Bloch form (7.66), then the ex­po­nen­tial will pro­duce the eigen­value dur­ing a shift. So the part $\pp{{\rm {p}},k}////$ must be the same af­ter the shift. Which means that it is pe­ri­odic of pe­riod $d$. (Note that you can al­ways write any wave func­tion in Bloch form; the non­triv­ial part is that $\pp{{\rm {p}},k}////$ is pe­ri­odic for ac­tual Bloch waves.)

If the crys­tal is in­fi­nite in size, the wave num­ber $k$ can take any value. (For a crys­tal in a fi­nite-size pe­ri­odic box as stud­ied in chap­ter 6.22, the val­ues of $k$ are dis­crete. How­ever, this sub­sec­tion will as­sume an in­fi­nite crys­tal.)

To un­der­stand what the Bloch form means for the elec­tron mo­tion, first con­sider the case that the pe­ri­odic fac­tor $\pp{{\rm {p}},k}////$ is just a triv­ial con­stant. In that case the Bloch waves are eigen­func­tions of lin­ear mo­men­tum. The lin­ear mo­men­tum $p$ is then ${\hbar}k$. That case ap­plies if the crys­tal po­ten­tial is just a triv­ial con­stant. In par­tic­u­lar, it is true if the elec­tron is in free space.

Even if there is a non­triv­ial crys­tal po­ten­tial, the so-called “crys­tal mo­men­tum” is still de­fined as:

$$\fbox{$\displaystyle p_{\rm cm} = \hbar k $} %$$ (7.67)

(In three di­men­sions, sub­sti­tute the vec­tors ${\skew0\vec p}$ and ${\vec k}$). But crys­tal mo­men­tum is not nor­mal mo­men­tum. In par­tic­u­lar, for an elec­tron in a crys­tal you can no longer get the prop­a­ga­tion ve­loc­ity by di­vid­ing the crys­tal mo­men­tum by the mass.

In­stead you can get the prop­a­ga­tion ve­loc­ity by dif­fer­en­ti­at­ing the en­ergy with re­spect to the crys­tal mo­men­tum, {D.45}:

$$\fbox{$\displaystyle v = \frac{{\rm d}{\vphantom' E}^{\rm p}}{{\rm d}p_{\rm cm}} \qquad p_{\rm cm} = \hbar k $} %$$ (7.68)

(In three di­men­sions, re­place the $p$-​de­riv­a­tive by 1$\raisebox{.5pt}{$/$}$​$\hbar$ times the gra­di­ent with re­spect to ${\vec k}$.) In free space, ${\vphantom' E}^{\rm p}$ $\vphantom0\raisebox{1.5pt}{$=$}$ $\hbar\omega$ and $p_{\rm {cm}}$ $\vphantom0\raisebox{1.5pt}{$=$}$ ${\hbar}k$, so the above ex­pres­sion for the elec­tron ve­loc­ity is just the ex­pres­sion for the group ve­loc­ity.

One con­clu­sion that can be drawn is that elec­trons in an ideal crys­tal keep mov­ing with the same speed for all times like they do in free space. They do not get scat­tered at all. The rea­son is that en­ergy eigen­func­tions are sta­tion­ary. Each eigen­func­tion cor­re­sponds to a sin­gle value of $k$ and so to a cor­re­spond­ing sin­gle value of the prop­a­ga­tion speed $v$ above. An elec­tron wave packet will in­volve a small range of en­ergy eigen­func­tions, and a cor­re­spond­ing small range of ve­loc­i­ties. But since the range of en­ergy eigen­func­tions does not change with time, nei­ther does the range of ve­loc­i­ties. Scat­ter­ing, which im­plies a change in ve­loc­ity, does not oc­cur.

This per­fectly or­ga­nized mo­tion of elec­trons through crys­tals is quite sur­pris­ing. If you make up a clas­si­cal pic­ture of an elec­tron mov­ing through a crys­tal, you would ex­pect that the elec­tron would pretty much bounce off every atom it en­coun­tered. It would then per­form a drunk­ard’s walk from atom to atom. That would re­ally slow down elec­tri­cal con­duc­tion. But it does not hap­pen. And in­deed, ex­per­i­men­tally elec­trons in met­als may move past many thou­sands of atoms with­out get­ting scat­tered. In very pure cop­per at very low cryo­genic tem­per­a­tures elec­trons may even move past many mil­lions of atoms be­fore get­ting scat­tered.

Note that a to­tal lack of scat­ter­ing only ap­plies to truly ideal crys­tals. Elec­trons can still get scat­tered by im­pu­ri­ties or other crys­tal de­fects. More im­por­tantly, at nor­mal tem­per­a­tures the atoms in the crys­tal are not ex­actly in their right po­si­tions due to ther­mal mo­tion. That too can scat­ter elec­trons. In quan­tum terms, the elec­trons then col­lide with the phonons of the crys­tal vi­bra­tions. The de­tails are too com­plex to be treated here, but it ex­plains why met­als con­duct much bet­ter still at cryo­genic tem­per­a­tures than at room tem­per­a­ture.

The next ques­tion is how does the prop­a­ga­tion ve­loc­ity of the elec­tron change if an ex­ter­nal force $F_{\rm {ext}}$ is ap­plied? It turns out that New­ton’s sec­ond law, in terms of mo­men­tum, still works if you sub­sti­tute the crys­tal mo­men­tum ${\hbar}k$ for the nor­mal mo­men­tum, {D.45}:

$$\fbox{$\displaystyle \frac{{\rm d}p_{\rm cm}}{{\rm d}t} = F_{\rm ext} \qquad p_{\rm cm} = \hbar k $} %$$ (7.69)

How­ever, since the ve­loc­ity is not just the crys­tal mo­men­tum di­vided by the mass, you can­not con­vert the left hand side to the usual mass times ac­cel­er­a­tion. The ac­cel­er­a­tion is in­stead, us­ing the chain rule of dif­fer­en­ti­a­tion,

$$\frac{{\rm d}v}{{\rm d}t} = \frac{{\rm d}^2{\vphantom' E}^... ...m d}^2{\vphantom' E}^{\rm p}}{{\rm d}p_{\rm cm}^2} F_{\rm ext}$$

For mass times ac­cel­er­a­tion to be the force, the fac­tor mul­ti­ply­ing the force in the fi­nal ex­pres­sion would have to be the rec­i­p­ro­cal of the elec­tron mass. It clearly is not; in gen­eral it is not even a con­stant.

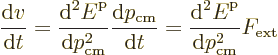
But physi­cists still like to think of the ef­fect of force as mass times ac­cel­er­a­tion of the elec­trons. So they cheat. They ig­nore the true mass of the elec­tron. In­stead they sim­ply de­fine a new “ef­fec­tive mass” for the elec­tron so that the ex­ter­nal force equals that ef­fec­tive mass times the ac­cel­er­a­tion:

$$\fbox{$\displaystyle m_{\rm eff} \equiv 1 \Bigg/ \frac{{\r... ...rm p}}{{\rm d}p_{\rm cm}^2} \qquad p_{\rm cm} = \hbar k $} %$$ (7.70)

Un­for­tu­nately, the ef­fec­tive mass is of­ten a com­pletely dif­fer­ent num­ber than the true mass of the elec­tron. In­deed, it is quite pos­si­ble for this mass to be­come neg­a­tive for some range of wave num­bers. Phys­i­cally that means that if you put a force on the elec­tron that pushes it one way, it will ac­cel­er­ate in the op­po­site di­rec­tion! That can re­ally hap­pen. It is a con­se­quence of the wave na­ture of quan­tum me­chan­ics. Waves in crys­tals can be re­flected just like elec­tro­mag­netic waves can, and a force on the elec­tron may move it to­wards stronger re­flec­tion.

For elec­trons near the bot­tom of the con­duc­tion band, the ef­fec­tive mass idea may be a bit more in­tu­itive. At the bot­tom of the con­duc­tion band, the en­ergy has a min­i­mum. From cal­cu­lus, if the en­ergy ${\vphantom' E}^{\rm p}$ has a min­i­mum at some wave num­ber vec­tor, then in a suit­ably ori­ented axis sys­tem it can be writ­ten as the Tay­lor se­ries

$${\vphantom' E}^{\rm p}= {\vphantom' E}^{\rm p}_{\rm min} +... ...artial^2{\vphantom' E}^{\rm p}}{\partial k_z^2} k_z^2 + \ldots$$

Here the wave num­ber val­ues are mea­sured from the po­si­tion of the min­i­mum. This can be rewrit­ten in terms of the crys­tal mo­menta and ef­fec­tive masses in each di­rec­tion as

$${\vphantom' E}^{\rm p}= {\vphantom' E}^{\rm p}_{\rm min} +... ...{1}{2}} \frac{1}{m_{{\rm eff},z}} p_{{\rm cm},z}^2 + \ldots %$$ (7.71)

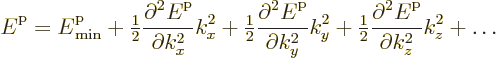
In this case the ef­fec­tive masses are in­deed pos­i­tive, since sec­ond de­riv­a­tives must be pos­i­tive near a min­i­mum. These elec­trons act much like clas­si­cal par­ti­cles. They move in the right di­rec­tion if you put a force on them. Un­for­tu­nately, the ef­fec­tive masses are not nec­es­sar­ily sim­i­lar to the true elec­tron mass, or even the same in each di­rec­tion.

For the ef­fec­tive mass of the holes at the top of a va­lence band things get much messier still. For typ­i­cal semi­con­duc­tors, the en­ergy no longer be­haves as an an­a­lytic func­tion, even though the en­ergy in a spe­cific di­rec­tion con­tin­ues to vary qua­drat­i­cally with the mag­ni­tude of the wave num­ber. So the Tay­lor se­ries is no longer valid. You then end up with such an­i­mals as heavy holes, light holes,” and “split-off holes. Such ef­fects will be ig­nored in this book.

Key Points

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The en­ergy eigen­func­tions for pe­ri­odic po­ten­tials take the form of Bloch waves, in­volv­ing a wave num­ber $k$.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The crys­tal mo­men­tum is de­fined as ${\hbar}k$.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The first de­riv­a­tive of the elec­tron en­ergy with re­spect to the crys­tal mo­men­tum gives the prop­a­ga­tion ve­loc­ity.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The sec­ond de­riv­a­tive of the elec­tron en­ergy with re­spect to the crys­tal mo­men­tum gives the rec­i­p­ro­cal of the ef­fec­tive mass of the elec­tron.